What are the Properties of Cyclic Quadrilaterals?

What are the Properties of Cyclic Quadrilaterals?

Cyclic quadrilateral

If all four points of a quadrilateral are on circle then it is called cyclic Quadrilateral.
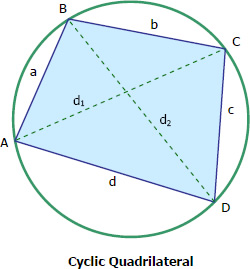A quadrilateral PQRS is said to be cyclic quadrilateral if there exists a circle passing through all its four vertices P, Q, R and S.
Let a cyclic quadrilateral be such that
PQ = a, QR = b, RS = c and SP = d.
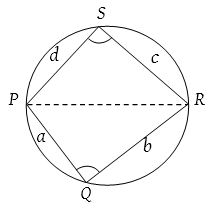Then ∠Q + ∠S = 180°, ∠A + ∠C = 180°
Let  2s = a + b + c + d

(1) Circumradius of cyclic quadrilateral: Circum circle of quadrilateral PQRS is also the circumcircle of ∆PQR.

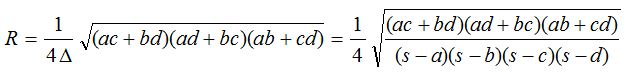
(2) Ptolemy’s theorem: In a cyclic quadrilateral PQRS, the product of diagonals is equal to the sum of the products of the length of the opposite sides i.e., According to Ptolemy’s theorem, for a cyclic quadrilateral PQRS.
PR.QS = PQ.RS + RQ.PS.

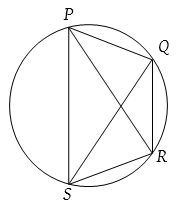
Properties of Cyclic Quadrilaterals

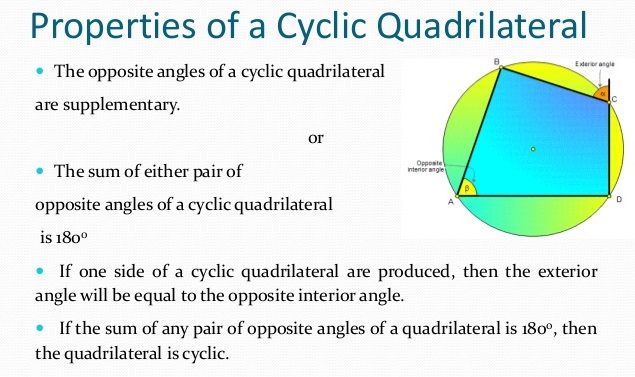
Theorem: Sum of opposite angles is 180º (or opposite angles of cyclic quadrilateral is supplementary)

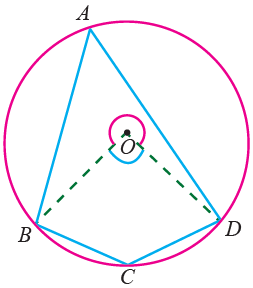
Given : O is the centre of circle. ABCD is the cyclic quadrilateral.
To prove : ∠BAD + ∠BCD = 180°, ∠ABC + ∠ADC = 180°
Construction : Join OB and OD
Proof:
(i) ∠BAD = (\(\frac { 1 }{ 2 } \))∠BOD.
(The angle substended by an arc at the centre is double the angle on the circle.)
(ii) ∠BCD = (\(\frac { 1 }{ 2 } \)) reflex ∠BOD.
(iii) ∠BAD + ∠BCD = (\(\frac { 1 }{ 2 } \))∠BOD + (\(\frac { 1 }{ 2 } \)) reflex ∠BOD.
Add (i) and (ii).
∠BAD + ∠BCD = (\(\frac { 1 }{ 2 } \))(∠BOD + reflex ∠BOD)
∠BAD + ∠BCD = (\(\frac { 1 }{ 2 } \)) × (360°)
(Complete angle at the centre is 360°)
∠BAD + ∠BCD = 180°
(iv) Similarly ∠ABC + ∠ADC = 180°.

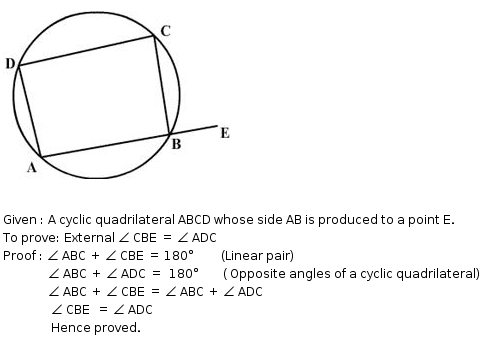
Exterior angle: Exterior angle of cyclic quadrilateral  is equal to opposite interior angle.

Read More: 

• Different Kinds of Quadrilateral
• More Solved examples on Quadrilaterals
• RS Aggarwal Class 9 Solutions Quadrilaterals and Parallelograms

Properties of Cyclic Quadrilaterals Example Problems With Solutions

Example 1:    Prove that the quadrilateral formed by the internal angle bisectors of any quadrilateral is cyclic.
Solution:

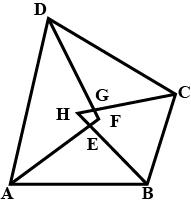
Given a quadrilateral ABCD with internal angle bisectors AF, BH, CH and DF of angles A, B, C and D respectively and the points E, F, G and H form a quadrilateral EFGH.
To prove that EFGH is a cyclic quadrilateral.
∠HEF = ∠AEB [Vertically opposite angles] ——– (1)
Consider triangle AEB,
∠AEB + \(\frac { 1 }{ 2 } \) ∠A + \(\frac { 1 }{ 2 } \) ∠ B = 180°
∠AEB = 180° – \(\frac { 1 }{ 2 } \) (∠A + ∠ B) ——– (2)
From (1) and (2),
∠HEF = 180° – \(\frac { 1 }{ 2 } \) (∠A + ∠ B) ——— (3)
Similarly, ∠HGF = 180° – \(\frac { 1 }{ 2 } \) (∠C + ∠ D) ——– (4)
From 3 and 4,
∠HEF + ∠HGF = 360° – \(\frac { 1 }{ 2 } \) (∠A + ∠B + ∠C + ∠ D)
= 360° – \(\frac { 1 }{ 2 } \) (360°)
= 360° – 180°
= 180°
So, EFGH is a cyclic quadrilateral since the sum of the opposite angles of the quadrilateral is 180°

Example 2:    ABCD is a cyclic quadrilateral whose diagonals intersect at a point E. If ∠DBC = 70º, ∠BAC is 30º, Find ∠BCD. Further, if AB = BC, find ∠ECD.
Solution:

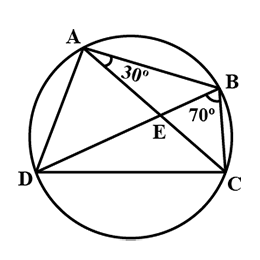
For chord CD,
∠CBD = ∠CAD … Angles in same segment
∠CAD = 70°
∠BAD = ∠BAC + ∠CAD = 30° + 70° = 100°
∠BCD + ∠BAD = 180° …Opposite angles of a cyclic quadrilateral
⇒ ∠BCD + 100° = 180°
⇒ ∠BCD = 80°
In △ABC
AB = BC (given)
∠BCA = ∠CAB … Angles opposite to equal sides of a triangle
∠BCA = 30°
Also, ∠BCD = 80°
∠BCA + ∠ACD = 80°
⇒ 30° + ∠ACD = 80°
∠ACD = 50°
∠ECD = 50°

Example 3:    Prove that a cyclic parallelogram is a rectangle.
Solution:

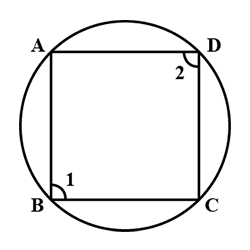
Given that, ABCD is a cyclic parallelogram.
To prove, ABCD is a rectangle.
Proof:
∠1 + ∠2 = 180° …Opposite angles of a cyclic parallelogram
Also, Opposite angles of a cyclic parallelogram are equal.
Thus,
∠1 = ∠2
⇒ ∠1 + ∠1 = 180°
⇒ ∠1 = 90°
One of the interior angle of the parallelogram is right angled. Thus, ABCD is a rectangle.

The post What are the Properties of Cyclic Quadrilaterals? appeared first on A Plus Topper.

from A Plus Topper
via Learning Made Simple 360

*Note that these contents are Autoblogged from A Plus Topper and cannot be edited.