Exponential Series

Exponential Series

Exponential Series

Definition (The number e)
The limiting value of \({ \left( 1+\frac { 1 }{ n }  \right)  }^{ n }\) when n tends to infinity is denoted by e.

Properties of e

(1) e lies between 2.7 and 2.8. i.e., 2.7 < e < 2.8.

(2) The value of e correct to 10 places of decimals is 2.7182818284.
(3) e is an irrational (incommensurable) number.
(4) e is the base of natural logarithm (Napier logarithm) i.e., ln x = log_e x and log₁₀ e is known as Napierian constant. log₁₀ e = 0.43429448, ln x = 2.303 log₁₀ x

Expansion of exponential series

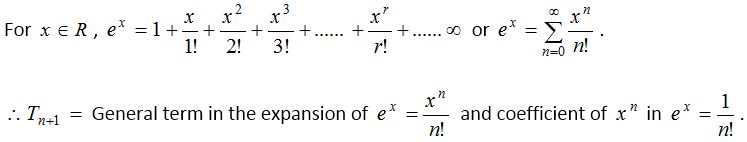
The above series known as exponential series and is called exponential function. Exponential function is also denoted by exp. i.e., e^x  exp A = e^A; ∴ exp x = e^x.
Replacing x by -x, we obtain

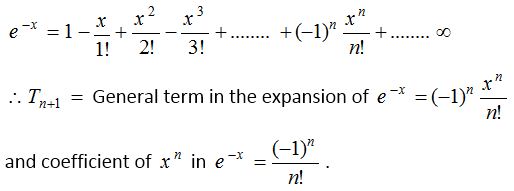
Exponential function a^x, where a > 0

Some standard results from exponential series

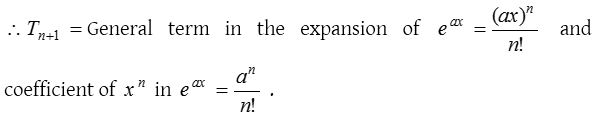
The post Exponential Series appeared first on A Plus Topper.

*Note that these contents are Autoblogged from A Plus Topper and cannot be edited.